Multiplication (Column Method)
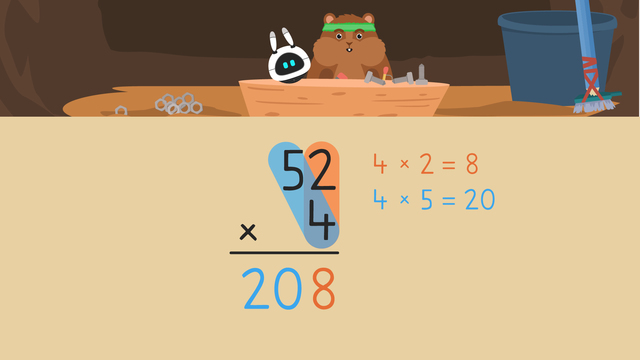

Basics on the topic Multiplication (Column Method)
Short Multiplication
In this video, Mr. Squeaks decides to make a new invention out of spare parts. When he turns it on there's just one problem, all it displays is multiplication equations! If you don’t how to do multiplication using short method multiplication, don’t worry! This video on column method for multiplication explains what is standard algorithm in multiplication, and how to do multiplication column method. Let's help Mr. Squeaks unlock his invention by practising short and long multiplication!
Multiplication Column Method
What is the column method in multiplication? When we want to answer the question, how do you do long and short multiplication? We need to first understand the column method. We can solve addition, subtraction and multiplication problems using different column methods. Solving multiplication with the columns method is a step-by-step way to solve a multiplication problem. We can use it to multiply by 1 digit and 2 digit numbers, or even more. Read on to find out how to find the answer to the question: how do you do long and short multiplication?
Multiplication Column Method – Example
Let’s look at some examples to find out how to do short multiplication using column method. Let’s take a look at a column method multiplication example so we can see how to do multiplication column method.
In this short multiplication example, we will be multiplying fifty-two times four.
Before we can do multiplication using column method, we must set up the problem by placing the larger factor on top, and line up the other factor below it. We must line up all the digits by place value, and include a multiplication symbol.

In order to calculate fifty-two times four first, multiply the bottom factor by the ones place, regrouping as needed. Four times two is eight, so we write it in the ones place.


Multiplication Column Method – Summary
Remember, long multiplication column method is a way of using the column method to solve a multiplication by 2 or more digits. Using the multiplication column method for short multiplication means multiplying by 1 digit.
Here are the steps for how to do multiplication column method:
| Step # | What to do? |
|---|---|
| 1 | Set up the equation vertically by lining up the place values. |
| 2 | Multiply the bottom factor by the ones place, regrouping as needed. |
| 3 | Multiply the bottom factor by the tens place, regrouping as needed. |
| 4 | Repeat the pattern as needed until you have multiplied all the place values. |
Follow these simple steps if you want to practise multiplication with standard algorithm!
If you want some more practise, you can find column multiplication worksheets to follow on from this multiplication using column method video, where you can practise this short multiplication method as well as activities to practise long multiplication column method. There are also interactive exercises and more activities waiting for you!
Transcript Multiplication (Column Method)
One afternoon long long ago, Mr. Squeaks decided to make a new invention out of spare parts. There's just one problem, all it displays is multiplication equations! Let's help Mr. Squeaks unlock his invention by multiplying up to three digits using the column method. The first equation we need to solve to help Mr. Squeaks is fifty-two times four. When we multiply numbers using the column method we place the larger number on top, and line up the other number below it. We must line up all the digits by place value, and include a multiplication symbol. In order to calculate fifty-two times four first, multiply the bottom factor by the ones place. Four times two is eight so we write it in the ones place here. Next move on to the next column. What is the product of four times five tens? Twenty tens, and we write it here. The product of fifty-two times four is two hundred and eight. Mr. Squeaks enters the product on the robot and another equation appears! Now, we'll calculate eighty-six times nine. First, set up the equation vertically by lining up the place values. Then, multiply the bottom factor by the ones place. What is the product of nine times six? Fifty four, so we write four in the ones column and a five in the tens column, as fifty ones is equal to five tens. Next, multiply the bottom factor by the tens place. What is the product of nine times eight tens? Seventy-two tens. Since we carried five tens to the tens place, we must add them on. Seventy-two tens plus five tens is seventy-seven tens. The product of eighty-six times nine is seven hundred and seventy-four. Mr. Squeaks enters the product on the robot, and one final equation appears! Lastly, we'll calculate two hundred and ninety-three times seven. First, set up the equation vertically by lining up the place values. Next, multiply the bottom factor by the ones place and make an exchange if the answer is two digits. What is the product of seven times three? Twenty-one, so we put one in the ones place and carry two tens here, as we can exchange twenty ones for two tens. Then, multiply the bottom factor by the tens place. What is the product of seven times nine tens? Sixty-three tens. Now, add the two tens we carried over after our exchange. Sixty-three plus two is sixty-five so we put five in the tens place and exchange our sixty tens for six hundreds, writing it in the hundred column. What do we do next? We multiply seven times two hundred to get fourteen hundreds. Then add on the six we carried over. Forteen plus six is twenty and we write it here. Remember to put a comma, starting after the ones and counting back three spaces. The product of two hundred and ninety-three times seven is two thousand and fifty-one! Remember when we multiply numbers up to three digits using the column method the first step is to set up the equation vertically by lining up the place values. The second step is to multiply the bottom factor by the ones place, making an exchange and carrying the digit over to the next column as needed. The third step is to multiply the bottom factor by the tens place, making an exchange if needed. The fourth step is to repeat the pattern until you have multiplied all the place values. Okay Mr. Squeaks, is your invention working? Mr. Squeaks has a new robot friend and it looks like he's called them Imani! What would you name his new friend? Write your name ideas in the comments below!
Multiplication (Column Method) exercise
-
Arrange the steps for solving a multiplication problem.
HintsThe first step is to set up the equation vertically by lining up the place values.
Multiply each place value starting with the ones place, regrouping when you need to.
Repeat the pattern until you have multiplied all the place values.
SolutionIn order to calculate 971 x 2:
- First, set up the equation vertically by lining up the place values of 971 and 2
- Second, multiply 2 x 1 = 2
- Then, multiply 2 x 7 = 14, regrouping the 1 to the hundreds place
- Now, multiply 2 x 9 = 18, and add 18 + 1 = 19
- Write the 19 below, which gives us the answer 1,942.
-
Which factors do we multiply first?
HintsFirst, multiply the ones place.
Next, multiply the tens place.
Solution- We highlight the 8 x 6 in yellow, since we multiply the ones place first.
- We highlight the 8 x 4 in blue, since we multiply the tens place second.
-
What is 38 x 5?
HintsFirst, multiply the bottom factor by the factor in the ones place.
In this illustration, you can see the steps for solving, starting with the orange highlight.
SolutionIn order to calculate 38 x 5:
- First, multiply the 5 x 8 = 40. Write 0 in the ones place, and regroup the 4 to the tens place
- Next, multiply 5 x 3 = 15
- Then, add 15 + 4 = 19
- Last, record 19, which gives us the answer 190.
-
What is 861 x 7?
HintsFirst, multiply 7 x 1.
Then, multiply 7 x 6. Last, multiply 7 x 8. Don't forget to regroup if you need to!
Solution- First multiply 7 x 1 = 7
- Next, multiply 7 x 6 = 42. Write the 2 in the tens place and regroup the 4 to the hundreds place
- Then, multiply 7 x 8 = 56. Add 56 + 4 = 60, which gives us the product 6,027.
-
What is 13 x 3?
HintsMultiply each place value, starting with the ones place.
Multiply 3 x 3, then multiply 3 x 1.
Solution- First, 3 x 3 = 9. Place a 9 in the equation on the right and in the product below.
- Next, multiply 3 x 1 = 3. Place a 3 in the equation on the right, and in the product below to get the final answer 39.
-
Complete each step for solving a multiplication equation.
HintsAlways start by multiplying the smallest place value on the right side of the equation, and work your way to the left.
Begin by multiplying the ones.
Solution- First, multiply the bottom number, 3, by the ones place, 8. 3 x 8 = 24. Write the 4 in the product, and regroup the 2 to the tens place.
- Next, multiply the bottom number, 3, by the tens place, 3. 3 x 3 = 9. Add 9 and the 2 we regrouped, which equals 11. Write the 1 in the product, and regroup the 1 to the hundreds place.
- Last, multiply the bottom number, 3, by the hundreds place, 4. 3 x 4 = 12. Add 12 and the 1 we regrouped, which equals 13. Write 13 in the space below to get the final product 1,314.
 Do you want to learn faster and more easily?
Do you want to learn faster and more easily?













